Hello, friend! Now that we have gone through the concept videos, the summary and the NCERT exercises, let us see how well we have understood the concepts of the chapter through this Practice Set. Try solving the questions on your own first using the summary, and if you get stuck you can always come here. I won’t tell anyone, I pinky promise!

Practice Problem 1
Determine the rational numbers \(p\) and \(q\) if \(\frac{7+\sqrt5}{7-\sqrt5}-\frac{7-\sqrt5}{7+\sqrt5}=p-7\sqrt5q.\)
Solution Let us first simplify the complicated-looking LHS using the LCM of the denominators, which is their product \((7-\sqrt5)(7+\sqrt5).\) \(\frac{7+\sqrt5}{7-\sqrt5}-\frac{7-\sqrt5}{7+\sqrt5}=\frac{(7+\sqrt5)(7+\sqrt5)-(7-\sqrt5)(7-\sqrt5)}{(7-\sqrt5)(7+\sqrt5)}=\frac{(7+\sqrt5)^2-(7-\sqrt5)^2}{(7-\sqrt5)(7+\sqrt5)}\) We use the identities \((a+b)^2=a^2+2ab+b^2 \) and \((a-b)^2=a^2-2ab+b^2\) in the numerator, and \((a+b)(a-b)=a^2-b^2\) in the denominator. \(\therefore \frac{7+\sqrt5}{7-\sqrt5}-\frac{7-\sqrt5}{7+\sqrt5}=\frac{{\color{red}{(}}7^2+2(7)(\sqrt5)+(\sqrt5)^2 {\color{red}{)}}-{\color{blue}{(}}~7^2-2(7)(\sqrt5)+(\sqrt5)^2 {\color{blue}{)}}}{(7-\sqrt5)(7+\sqrt5)}=\frac{49+14\sqrt5+5-49+14\sqrt5-5}{49-5}=\frac{28\sqrt5}{44}=\frac{7\sqrt5 \times4}{44}=\frac{7\sqrt5}{11}\) Thus, the initial equation can now be written as \(\frac{7\sqrt5}{11}=p-7\sqrt5q.\) \(\therefore \frac{7\sqrt5}{11}={\color{red}{0}}-7\sqrt5\times {\color{blue}{-\frac{1}{11}}}={\color{red}{p}}-7\sqrt5{\color{blue}{q}}\) Comparing both sides of the above equation, we get \(p=0\) and \(q=-\frac{1}{11}.\) If \(x=\frac{3-\sqrt{13}}{2},\) then find the value of \(x^2+\frac{1}{x^2}.\) Solution We have \(x=\frac{3-\sqrt{13}}{2}.—(1)\) \(\implies x^2=(\frac{3-\sqrt{13}}{2})^2=\frac{(3-\sqrt{13})^2}{2^2}=\frac{3^2-2(3)(\sqrt{13})+(\sqrt{13})^2}{4}=\frac{9-6\sqrt{13}+13}{4}=\frac{22-6\sqrt{13}}{4}=\frac{11-3\sqrt{13}}{2}\) (\(\because (a-b)^2=a^2-2ab+b^2\)) \(\therefore \frac{1}{x^2}=\frac{1}{(\frac{11-3\sqrt{13}}{2})}=\frac{2}{11-3\sqrt{13}}\) Combining \((1)\) and \((2),\) we have: \( x^2+\frac{1}{x^2}=\frac{11-3\sqrt{13}}{2}+\frac{2}{11-3\sqrt{13}}=\frac{(11-3\sqrt{13})(11-3\sqrt{13})+2(2)}{2(11-3\sqrt{13})}=\frac{(11-3\sqrt{13})^2+4}{2(11-3\sqrt{13})}=\frac{11^2-2(11)(3\sqrt{13})+(3\sqrt{13})^2+4}{2(11-3\sqrt{13})}\) \(\therefore x^2+\frac{1}{x^2}=\frac{121-66\sqrt{13}+117+4}{2(11-3\sqrt{13})}=\frac{242-66\sqrt{13}}{2(11-3\sqrt{13})}=\frac{22(11-3\sqrt{13})}{2(11-3\sqrt{13})}=\frac{22}{2}=11\) Thus, we have \(x^2+\frac{1}{x^2}=11.\) Classify the following numbers as rational or irrational: (i) \(0.2222\) (ii) \(0.2222…\) (iii) \(0.22228649123…\) (iv) \(1.5042056719362740\) Solution (i) \(0.2222\) The given decimal is terminating. Hence, it represents a rational number. (ii) \(0.2222…\) The given decimal is non-terminating and repeating. Thus, \(0.2222…=0.\overline{2}\) represents a rational number. (iii) \(0.22228649123…\) The given decimal is non-terminating and non-repeating, and hence represents an irrational number. (iv) \(1.5042056719362740\) The given decimal is terminating, and hence represents a rational number. If \(\sqrt{10+\sqrt{24}+\sqrt{60}+\sqrt{40}}=\sqrt{a}+\sqrt{b}+\sqrt{c},\) where \(a < b< c,\) then find the value of \(a+b-c.\) Solution Let us consider \(\sqrt{10+\sqrt{24}+\sqrt{60}+\sqrt{40}}.\) Replacing \(\color{red}{10}\) by \({\color{red}{2+3+5}},\) we get: \(\sqrt{{\color{red}{10}}+\sqrt{24}+\sqrt{60}+\sqrt{40}}=\sqrt{{\color{red}{2+3+5}}+\sqrt{24}+\sqrt{60}+\sqrt{40}}=\sqrt{2+3+5+2\sqrt6+2\sqrt{15}+2\sqrt{10}}\) \(=\sqrt{(\sqrt2)^2+(\sqrt3)^2+(\sqrt5)^2+2\sqrt2\sqrt3+2\sqrt3\sqrt5+2\sqrt2\sqrt5}\) \(=\sqrt{({\color{red}{\sqrt{2}}}+{\color{blue}{\sqrt{3}}}+{\color{pink}{\sqrt{5}}})^2}~ ~ ~ ~ ~ (\because {\color{red}{a}}^2+{\color{blue}{b}}^2+{\color{pink}{c}}^2+2{\color{red}{a}}{\color{blue}{b}}+2{\color{blue}{b}}{\color{pink}{c}}+2{\color{red}{a}}{\color{pink}{c}}=({\color{red}{a}}+{\color{blue}{b}}+{\color{pink}{c}})^2)\) \(=\sqrt2+\sqrt3+\sqrt5\) \(\therefore \sqrt{10+\sqrt{24}+\sqrt{60}+\sqrt{40}}=\sqrt2+\sqrt3+\sqrt5~ ~ —(1)\) But, we are given that \(\sqrt{10+\sqrt{24}+\sqrt{60}+\sqrt{40}}=\sqrt{a}+\sqrt{b}+\sqrt{c},\) where \(a<b<c.\) Thus, comparing with \((1),\) we get: \(a=2, b=3, c=5 ~ ~ ~ (\because a<b<c)\) \(\therefore a+b-c=2+3-5=5-5=0\) If \(x=9+4\sqrt5,\) then find the value of \(\sqrt{x}+\frac{1}{\sqrt{x}}.\) Solution We are given that \(x=9+4\sqrt5.\) \(\implies \sqrt{x}=\sqrt{\underline{9}+4\sqrt5}=\sqrt{\underline{5+4}+4\sqrt5}=\sqrt{({\color{red}{\sqrt5}})^2+({\color{blue}{2}})^2+2({\color{red}{\sqrt5}})({\color{blue}{2}})}\) \(= \sqrt{({\color{red}{\sqrt5}}+{\color{blue}{2}})^2}~ ~ (\because {\color{red}{a}}^2+{\color{blue}{b}}^2+2{\color{red}{a}}{\color{blue}{b}}=({\color{red}{a}}+{\color{blue}{b}})^2)\) \(\therefore \sqrt{x}=\sqrt5+2~ ~ ~ ~ —(1)\) \(\implies \frac{1}{\sqrt{x}}=\frac{1}{\sqrt5+2}=\frac{1}{\sqrt5+2} \times \frac{\color{red}{\sqrt5-2}}{\color{red}{\sqrt5-2}}=\frac{\sqrt5-2}{(\sqrt5+2)(\sqrt5-2)}=\frac{\sqrt5-2}{(\sqrt5)^2-(2)^2}~ ~ ~ (\because (a+b)(a-b)=a^2-b^2)\) \(\therefore \frac{1}{\sqrt{x}}=\frac{\sqrt5-2}{5-4}=\frac{\sqrt5-2}{1}=\sqrt5-2~ ~ ~ ~ —(2)\) \(\therefore (1)\text{ and }(2)\implies \sqrt{x}+\frac{1}{\sqrt{x}}=\sqrt5+2+\sqrt5-2=2\sqrt5\)Practice Problem 2
Practice Problem 3
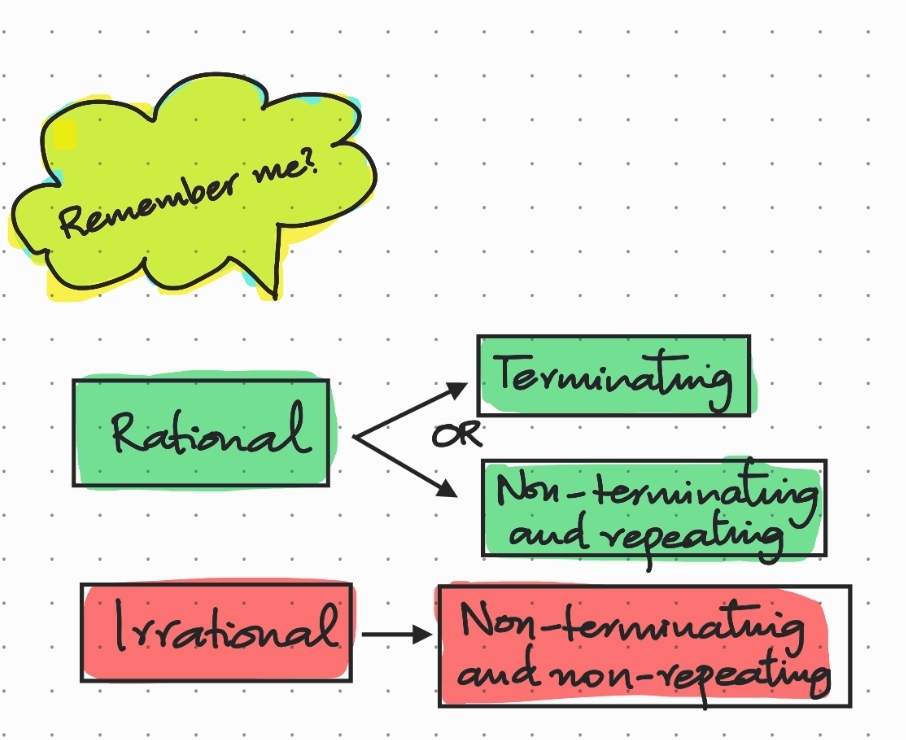
Practice Problem 4
Practice Problem 5