Simplify the following expression:
\(\dfrac{-2pl^2}{3yz}\div\dfrac{9lp^2}{5xy}\)
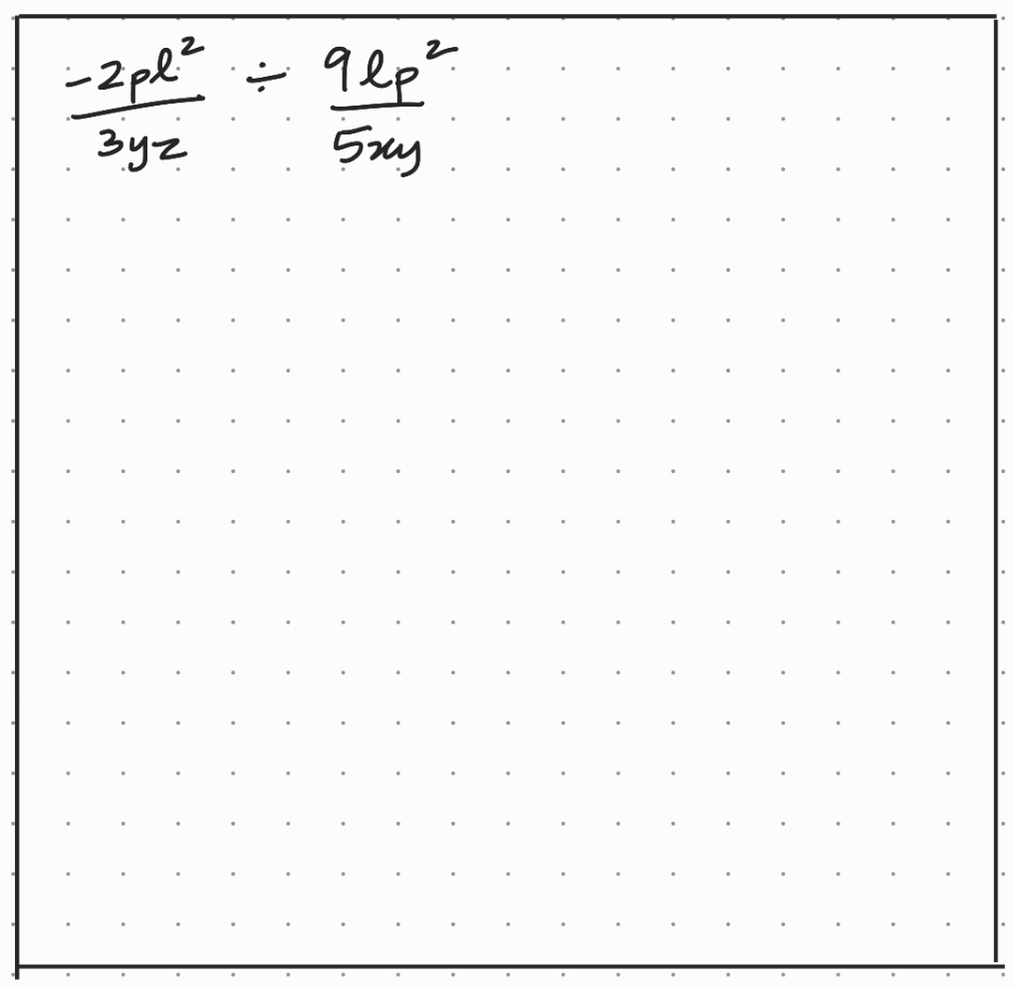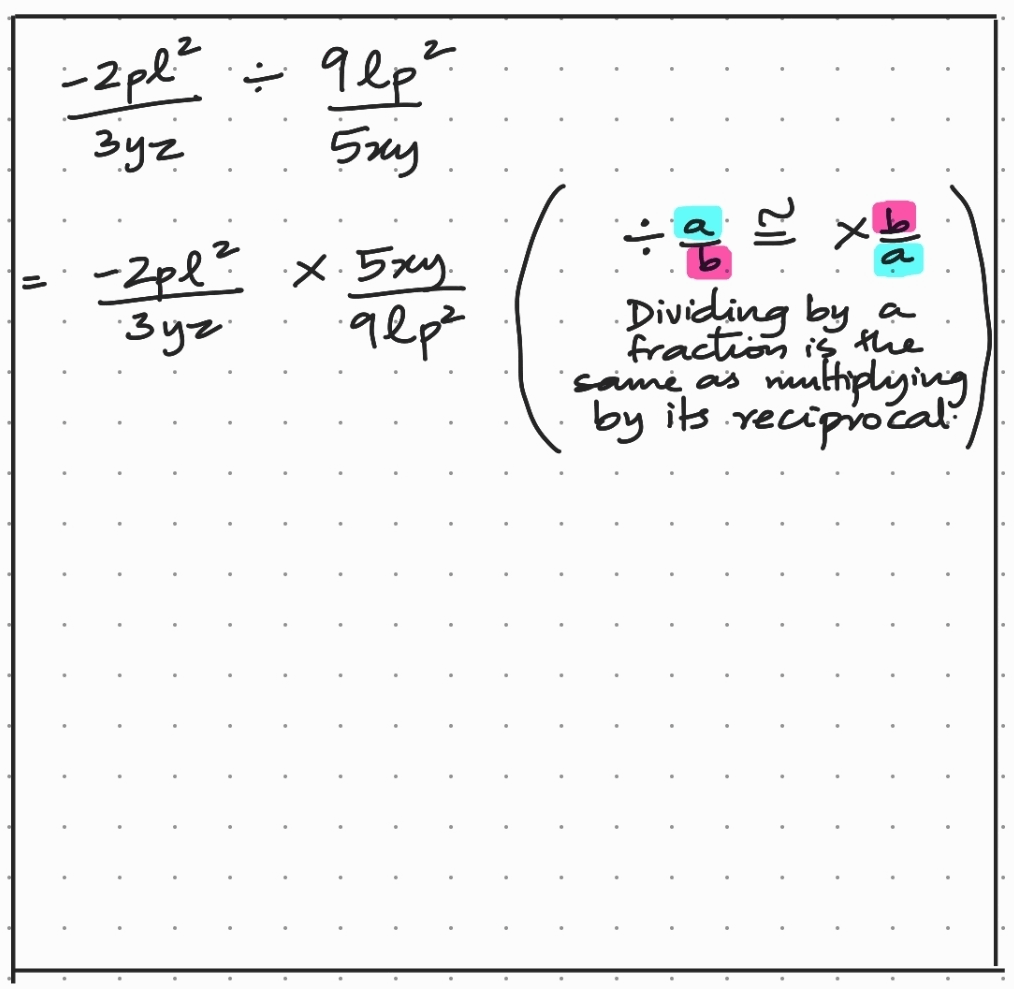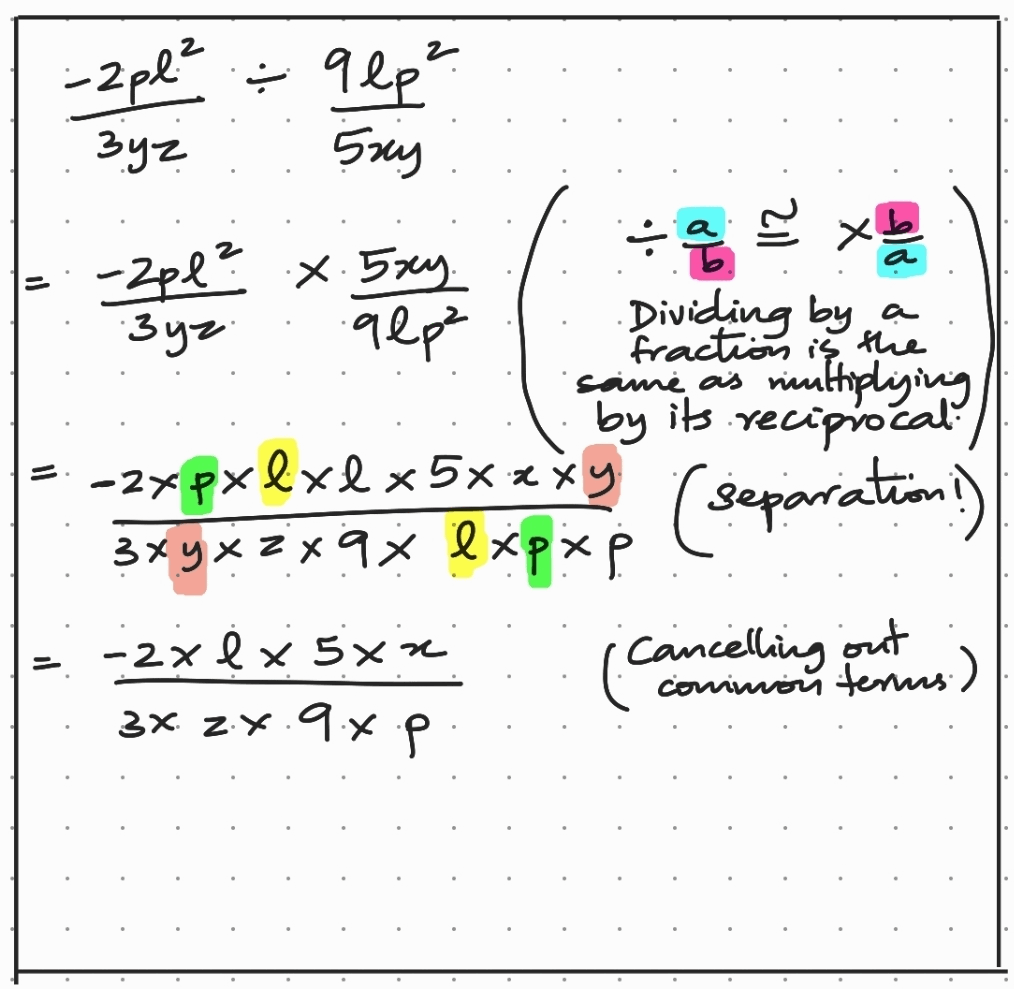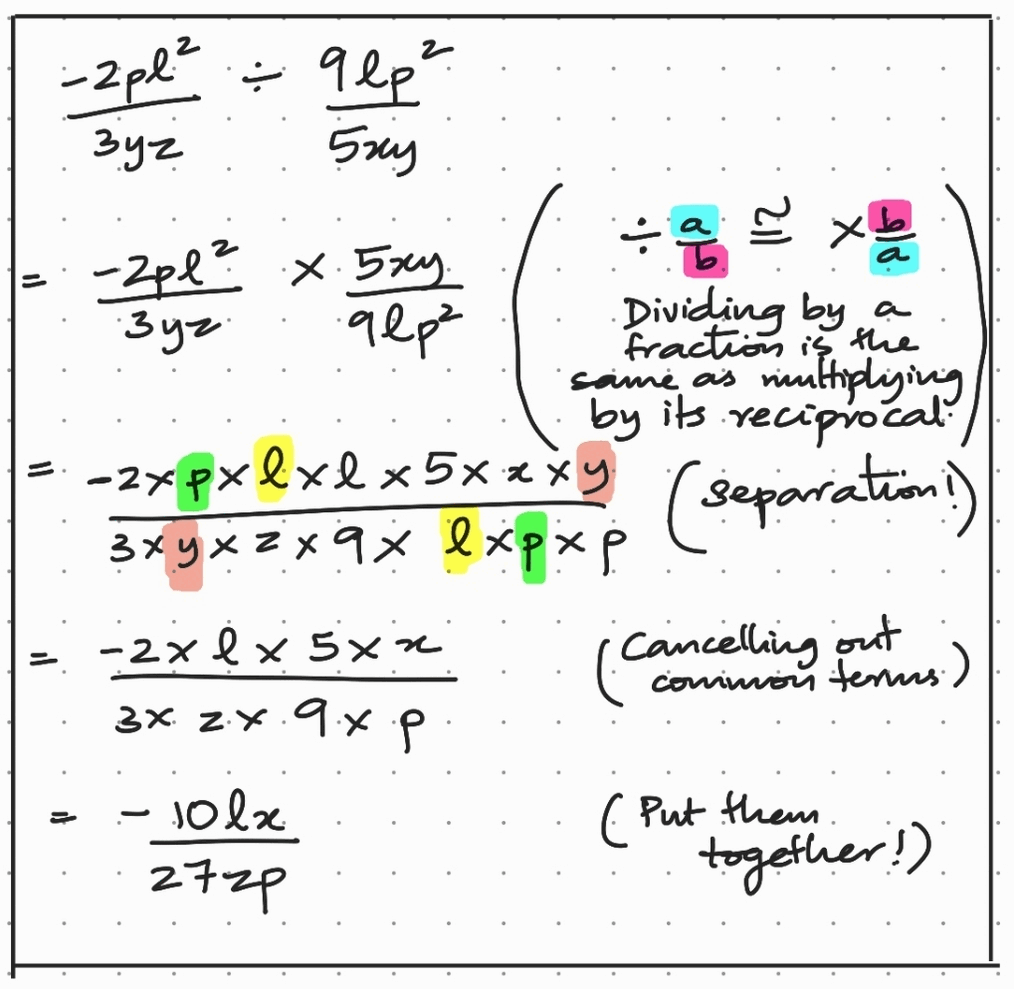
Detailed look at the solution:
Let us consider the given expression.
\(\dfrac{-2pl^2}{3yz}\div\dfrac{9lp^2}{5xy}=\dfrac{-2pl^2}{3yz} \times \dfrac{5xy}{9lp^2}=\dfrac{-2 \times p \times l \times l \times5 \times x \times y}{3 \times y \times z \times 9 \times l \times p \times p}=\dfrac{-2\times l\times 5 \times x}{3\times z \times 9\times p}=-\dfrac{10lx}{27zp}\)
Note that we have used the following rule in simplifying the given expression:
Dividing a fraction \(\dfrac{a}{b}\) by a fraction \(\dfrac{c}{d}\) is the same as multiplying \(\dfrac{a}{b}\) by the reciprocal of \(\dfrac{c}{d},\) which is \(\dfrac{d}{c}.\)
In other words, we have \(\dfrac{a}{b} \div \dfrac{c}{d}=\dfrac{a}{b} \times \dfrac{d}{c}.\)
Double note that (I like the phrase! I am going to keep it!) the reciprocal of \(\dfrac{c}{d}\) is given by \(\dfrac{1}{\dfrac{c}{d}}=\dfrac{d}{c}.\)